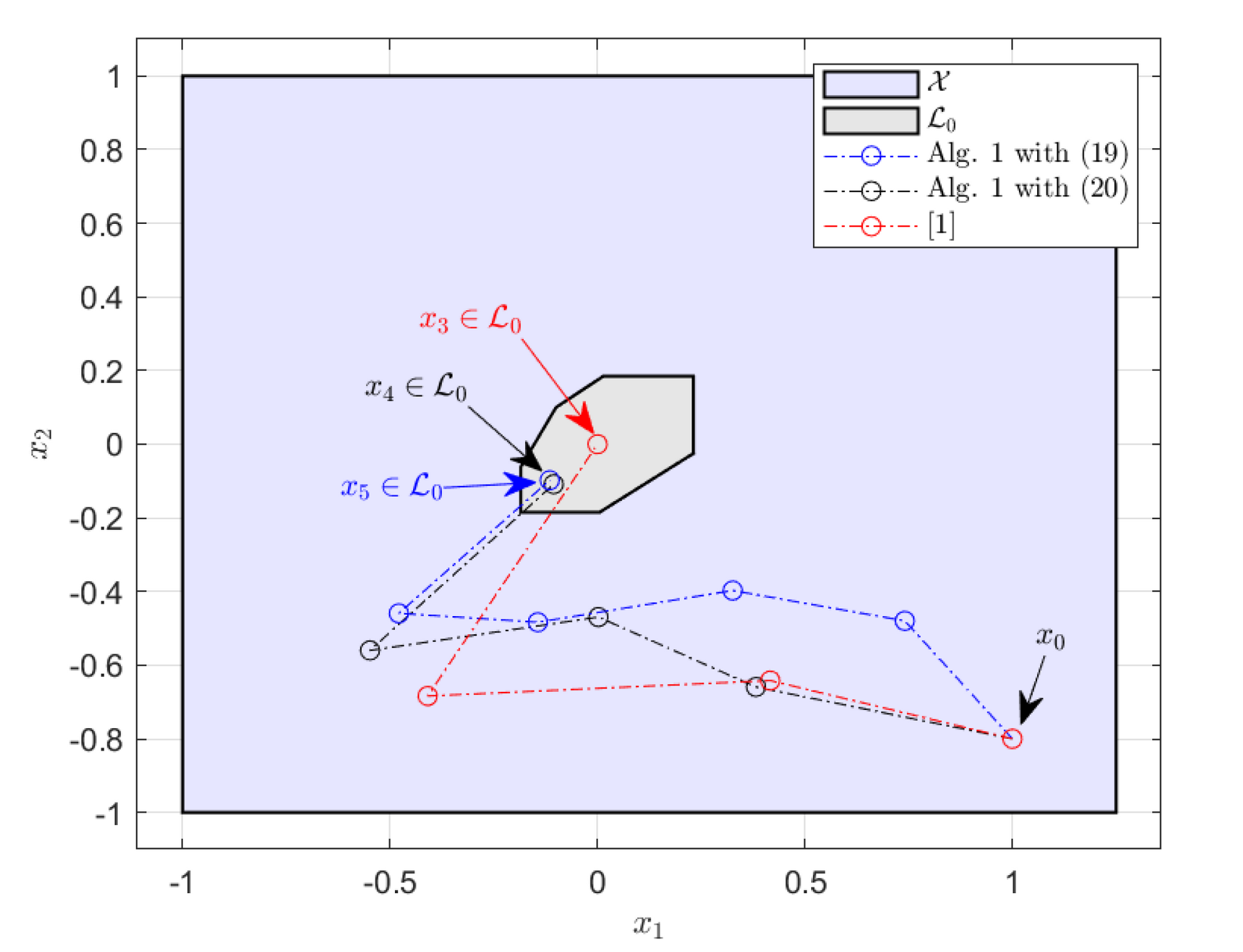
This paper addresses the problem of designing output feedback controllers for constrained linear systems subject to bounded process and measurement disturbances. The proposed solution extends the set-theoretic model predictive control framework to deal with constrained output regulation problems. In particular, this is here achieved by adequately exploiting the extended Farka’s lemma to offline compute through bilinear optimization problems, a family of robust one-step controllable sets, and associated output feedback control gains. It is then formally proved that such computations can be online leveraged to design a simple switching controller capable of ensuring, by construction, that the state-trajectory of the system is always uniformly ultimately bounded, in a finite number of steps, into a small robust control invariant region. Finally, the effectiveness and benefits of the proposed solution are verified through a numerical example and compared with three alternative schemes.

W. Lucia,  J. G. Ernesto,  E. B. Castelan, "Set-theoretic output feedback control: a bilinear programming approach," Automatica, 2023